Las funciones vienen dadas implícita o implícitamente, por ejemplo, en el siguiente modelo matemático no tenemos expresada explícitamente la función y=f(x), es decir, no tenemos expresada a ''y'' en función de x, lo ideal es que esto ocurra, es por ello que vamos a hacer un procedimiento para lograr esto.
The functions are given implicitly or implicitly, for example, in the following mathematical model we do not have explicitly expressed the function y=f(x), that is to say, we do not have ''y'' expressed as a function of x, the ideal is that this happens, that is why we are going to make a procedure to achieve this.
Despejamos x de la ecuación:// We clear x from the equation:
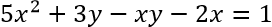
Nos queda así:// We are left with the following:
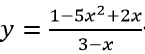
Al despejar la y se puede obtener la derivada y^' sin ninguna dificultad aplicando la derivada de un cociente, dicha técnica de derivación, esta explicada en este post [1].
By clearing y, the derivative y’ can be obtained without any difficulty by applying the derivative of a quotient, this derivative technique is explained in this post[1].
Al realizarlo, nos queda así:// When this is done, it looks like this:
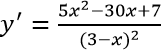
Es decir que hemos obtenido la derivada de y de la manera convencional.// That is, we have obtained the derivative of y in the conventional way.
Pero existe una forma de obtener la derivada de y, sin despejarla de su expresión implícita, tal técnica se denomina ‘’Derivación Implícita’’ y es lo que vamos a desarrollar hoy en este post.
But there is a way to obtain the derivative of y, without clearing it from its implicit expression, such technique is called ''Implicit Derivative'' and it is what we are going to develop today in this post.
Comencemos// Let's start
El objetivo de este post es explicar la técnica que permite derivar a una función implícitamente, particularmente con el ejemplo inicialmente planteado.
The aim of this post is to explain the technique that allows to derive a function implicitly, particularly with the example initially given.
Es decir:// That is to say:
Hallar y' derivando implícitamente la expresión:// Find y' by implicitly deriving the expression:

Para ello, se aplican todas la técnicas de derivación vistas anteriormente, y en especial la regla de la cadena que nos da fórmula para derivar y^n, es decir:
To do so, we apply all the derivation techniques seen above, and especially the chain rule that gives us the formula to derive y^n, that is:
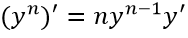
Procedamos entonces:// Let us proceed then:
Lo primero que vamos a hacer, es derivar a ambos miembros de la igualdad:// The first thing we are going to do, is to derive both members of the equality:
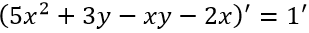
Luego aplicamos la técnica de suma y diferencia de funciones (vea post[2]):// Then we apply the technique of sum and difference of functions (see post[2]):
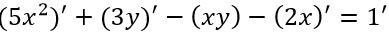
Aquí derivamos término a término:// Here we derive term by term:
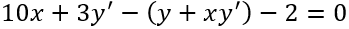
Eliminando el paréntesis nos queda así:// Eliminating the parenthesis we are left with the following:
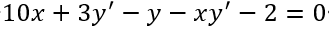
Pasamos 10x, y , también -2 al segundo miembro de la igualdad así:// We pass 10x, y , also -2 to the second member of the equality as follows:
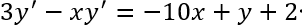
Sacamos factor común y^' en el lado izquierdo de la igualdad así:// We take out the common factor y' on the left side of the equality as follows:
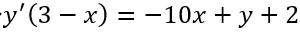
Despejando no queda que: // Clearing does not leave that:
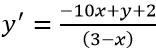
Lo cual es el resultado esperado. // This is the expected result.
Aparentemente, este resultado difiere de la derivada y^' obtenido en la derivada explicita obtenida anteriormente, pero esto no es así; aquí lo que hace falta es una comprobación de que ambas son iguales, y eso se hace sustituyendo:
Apparently, this result differs from the derivative y^' obtained in the explicit derivative obtained above, but this is not so; here what is needed is a check that both are equal, and that is done by substituting:
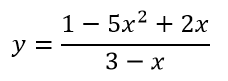
En // At
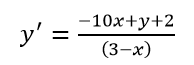
Así: // Thus:

Con lo cual se verifica la equivalencia de los dos resultados. // This verifies the equivalence of the two results.
A continuación, dejo un ejercicio planteado para el lector: // Here is an exercise for the reader:
Derivando implícitamente, hallar la derivada de: // Deriving implicitly, find the derivative of:
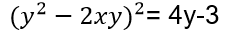
Solución // Solution
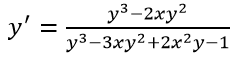
Recordar que: // Remember that:
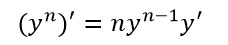
Cualquier duda puede ser planteada a través de los comentarios. // Any questions can be raised in the comments.

Referencias//References
El texto es original de la autora, la imagen es de Pixabay.///The text is original by the author.The image is from Pixabay.[1]https://hive.blog/cervantes/@analealsuarez/tecnicas-de-derivacion-derivada-de-un-cociente-de-funciones-derivative-techniques-derivative-of-a-quotient-of-functions
[2]https://hive.blog/cervantes/@analealsuarez/tecnicas-de-derivacion-derivada-de-una-suma-de-funciones
Su publicación ha sido votada por @Edu-venezuela, se trasladará a otros proyectos de curación para obtener más apoyo. ¡Sigan con el buen trabajo!
Muchísimas gracias.
Calculous is 🆒😎. Good to see you put it here.
Muchísimas gracias.