A post by @gavvet entitled Is there a scientific basis for Jesus Christ? put forward the idea that sin can be defined as something that moves people into a state of disorder. I find this a fascinating rationale to explore, as @gavvet did. However, as I read that post, I was reminded of the Mandelbrot Set, a set of numbers that, when iterated, becomes what some mathematicians call the "thumbprint of God." This took my mind in a somewhat different direction.
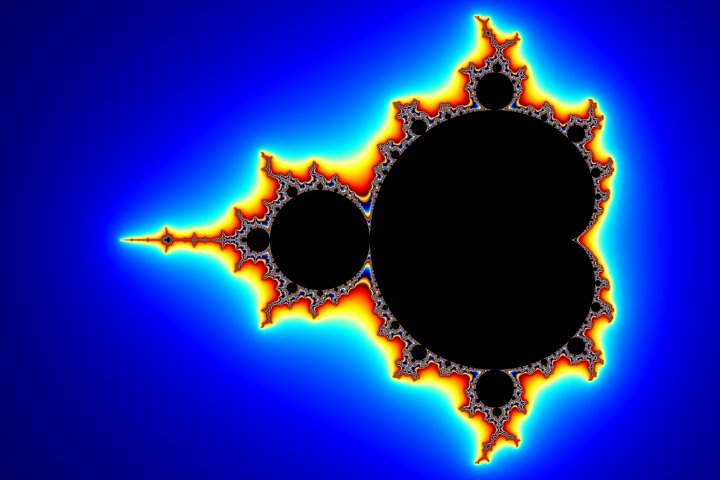
The Mandelbrot Set is described as an infinitely complex pattern generated from a very simple rule: z = z2 + c, and its iterated expression creates a profound and beautiful image that is infinitely complex. To quote Arthur C. Clarke, regarding how the M-Set is generated:
"When the Mandelbrot equation is given a number, representing a point, and that number is iterated through the equation, one of two things happen. Either the number gets bigger and bigger, shoots off to infinity, or it shrinks to zero. Depending on which happens, the computer then knows where to draw a boundary line. So what we get from this basic iteration is a kind of map dividing this world into two distinct territories. Outside of it are all the numbers that have the freedom of infinity. Inside it, numbers that are prisoners, trapped and doomed to ultimate extinction."
The numbers shooting off to infinity appear on the M-Set boundary and are colored according to how fast they move out toward infinity, while the numbers descending to zero are colored black.
Returning to @gavvet's definition of sin, there is no disorder in the M-set, so no "sin" per se. But I'd like to apply another definition of sin, that being anything that moves a person away from God. Using that definition, sin could exist in the M-Set in all equations where the iterations of a number move it toward zero. Sin, in this context, comprises all the black areas, while numerical movements toward God, all those equations and iterations without "sin," are expressed as the beautifully colored boundary elements. Might this offer a mathematical basis for understanding the concept of sin? I would love to hear others' thoughts, and it's my hope @gavvet will chime in, as well.
@daoine-sidhe is the author of Galloglass, a novel in progress that addresses the somewhat decentralized nature of medieval Ireland in the context of highly centralized empires like England. Brighid, the faery poet and time-traveling Keynesian, sparks a war that illustrates these differences in centralization. (Brighid is helped in her desire to spark war by John de Courcy's mint in Carrikfergus, which irritates the English crown and provides a great launching pad for a poetic discussion of currency, inflation, and debt.) It also illustrates the way a war is funded and why it appeals so much to Keynesians. Galloglass: Chapter 1 is available on Steemit -- please give it a glance and leave me your feedback! (And be brutal; I can take it. In fact, I need it.) Sláinte!
Liked the post, just not sure it's a valid logical argument. That being said, it was an interesting conclusion and one which has me thinking. Have an upvote for good measure and I plan to follow. I have featured your post on my daily hidden gems blog entry. Thanks for the great content and I will be looking out for more.
Yeah, I'm not sure it's a valid logical argument, either. I'm still thinking on it, too. Thank you very much for including me on your hidden gems!!! That's awesome. And I'm happy to reciprocate the follow.
What if God is zero and not infinity? If infinity, he/she/it can never be reached.
A sin is an incorrect proof.
This post has been linked to from another place on Steem.
Learn more about linkback bot v0.3
Upvote if you want the bot to continue posting linkbacks for your posts. Flag if otherwise. Built by @ontofractal