Another application of the integral is to calculate the length of a curve segment, taking into account that an arc is a curve segment, as shown in the following figure:

Commonly we have calculated the distance to a line segment, but the problem arises when we try to calculate the distance to a curve segment, since we have to make an approximation, this approximation is done by applying an application that has the integral by the definition of arc length.
Definition of arc length.
Let y = f(x) represent a smooth curve in the interval [a,b] the arc length of f between a and b is:
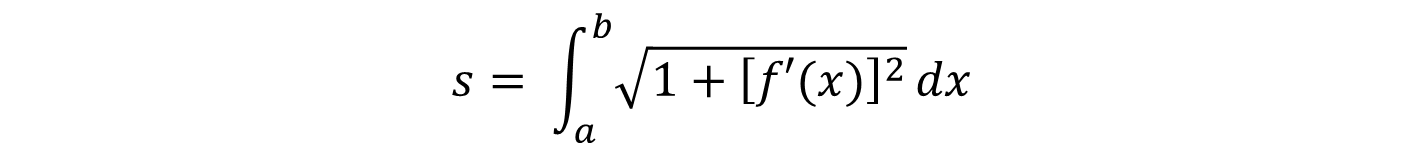
The formula for calculating the arc length of a smooth curve between a and ab is only when the function is of the form y = f(x), because if the function changes to the form x = g(y) then the interval changes, but this case we will only assume when the curve is represented by a function of the form y = f(x).
Application in the use of the integral to calculate the arc length of a smooth curve
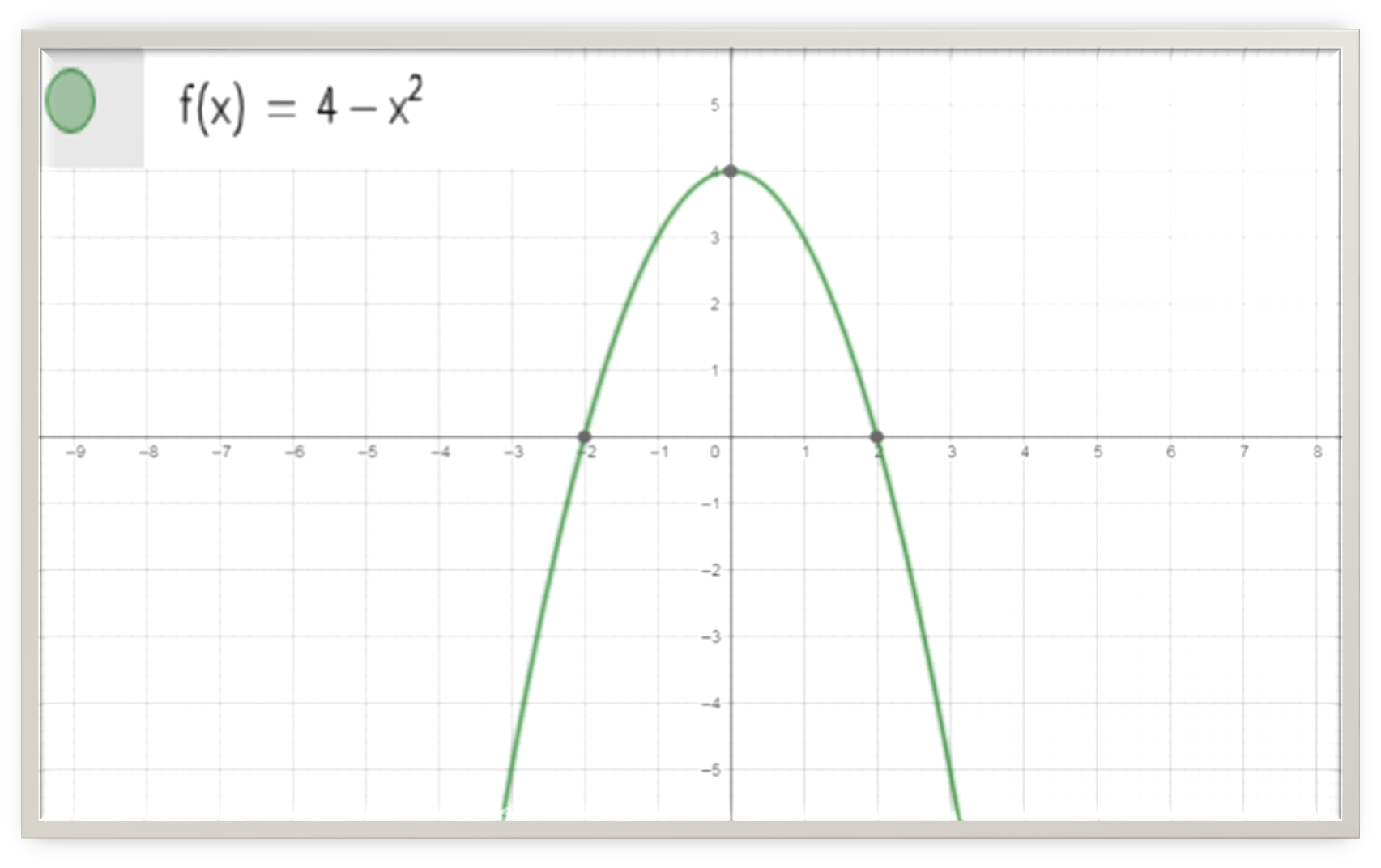
Calculate the arc length of the curve representing f(x) = 4 -x2 in the interval (0,2).
The first thing is to find the curve representing the parabolic function in the interval of (0,2):
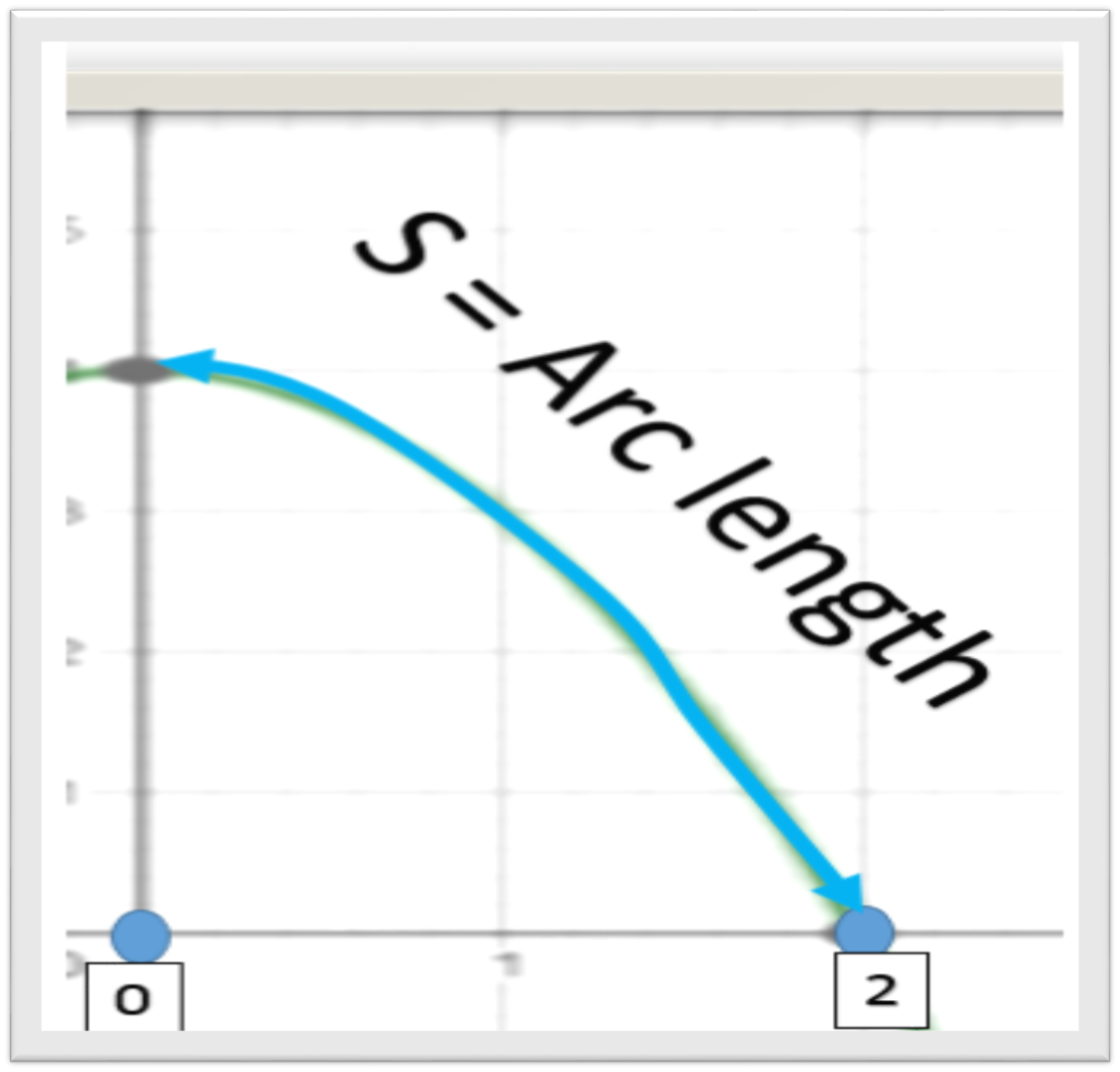
If we take into account that of the parabolic function, the arc length is only the portion that goes from zero to two, then the arc length is:
Then we consider the formula for calculating the arc length:
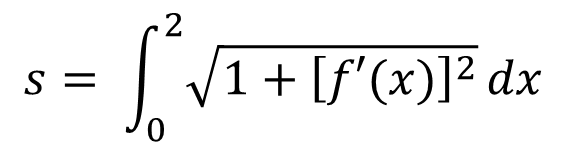
As you can see we need the derivative of the parabolic function:

Substitute f'(x) in the formula for arc length calculation:

Finally, we are left with the integral approach:
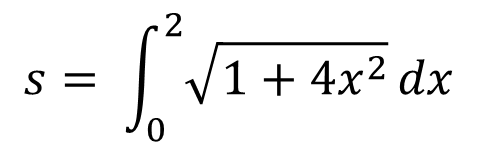
We solve the definite integral:
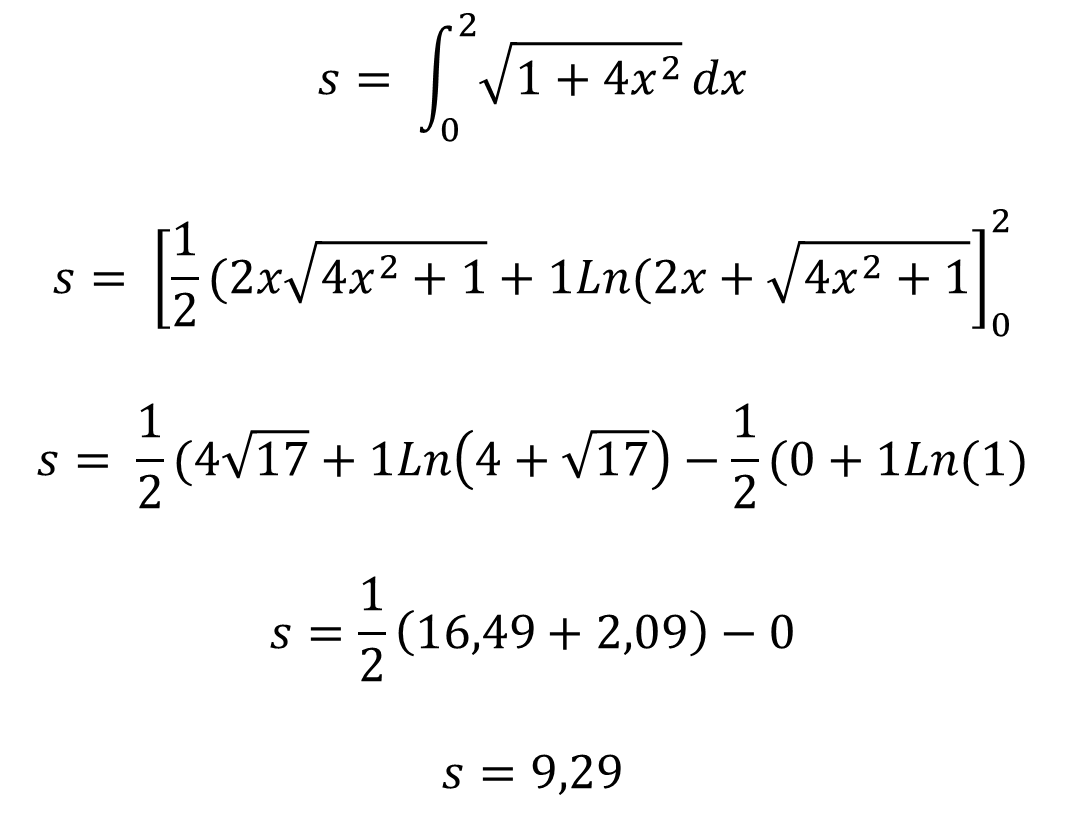
The arc length of the curve segment that goes from zero to 2 is 9.29.
The meaning and learning of calculating the arc length is that we know that we have to apply another mathematical artifice different from the calculation of the distance between two points, since a straight line passes through two points, but the length to be calculated is not that of a straight line, but to calculate the length of a curve segment, and for this we use the integral as an application tool.
Bibliographic reference consulted and recommended
Book of calculus with analytic geometry by Larson and Hostetler. Volume I. 8th edition.
Note: All images are my own and were elaborated using Microsoft PowerPoint design tools and GeoGebra software.
wonderful exercise! What did you use to write the various formulas? I use a digital tablet, but your writing is definitely better
Hello friend @stefano.massari.
I do the equations in Microsoft PowerPoint.
I give insert equations, and I use the tools that insert equations gives me.
Microsoft Word also provides the same tools.
Greetings friend, I hope you can experiment with this way of doing the equations.
Thanks for replying. I will look for a way to do equations with microsoft word