Hello friends and followers of STEM content, this time I want to share with you how we can calculate the relative extrema (maximum point and minimum point) of a cubic function by applying the criterion of the first derivative.
To do this the proposal is to find the maximum and minimum point of the following cubic function:

Why is it necessary to use the criterion of the first derivative to know the maximum and minimum points of the cubic function?
The cubic function will have a behavior where it grows and reaches the highest point and then decreases and reaches the lowest point and then grows indefinitely to infinity. Logically we find the images of the function, i.e. we give arbitrary values to the variable x to know its image f(x).
However with these values of the images we can be left inconclusive the cuts with the coordinate axes X and Y, and also we are left inconclusive the coordinates of the maximum and minimum points of the cubic function.
If we use a software that allows us to introduce the equation of the cubic function we will be able to know the cuts with the coordinate axes and the coordinates of the maximum and minimum points, we will also be able to deduce its behavior. For this case I have used the software geogebra in its last version, when we open the application we see something like this:
Then we enter the equation of the cubic function using the digital keyboard tools displayed by the program:
The graph is then displayed on the right side, as shown below:
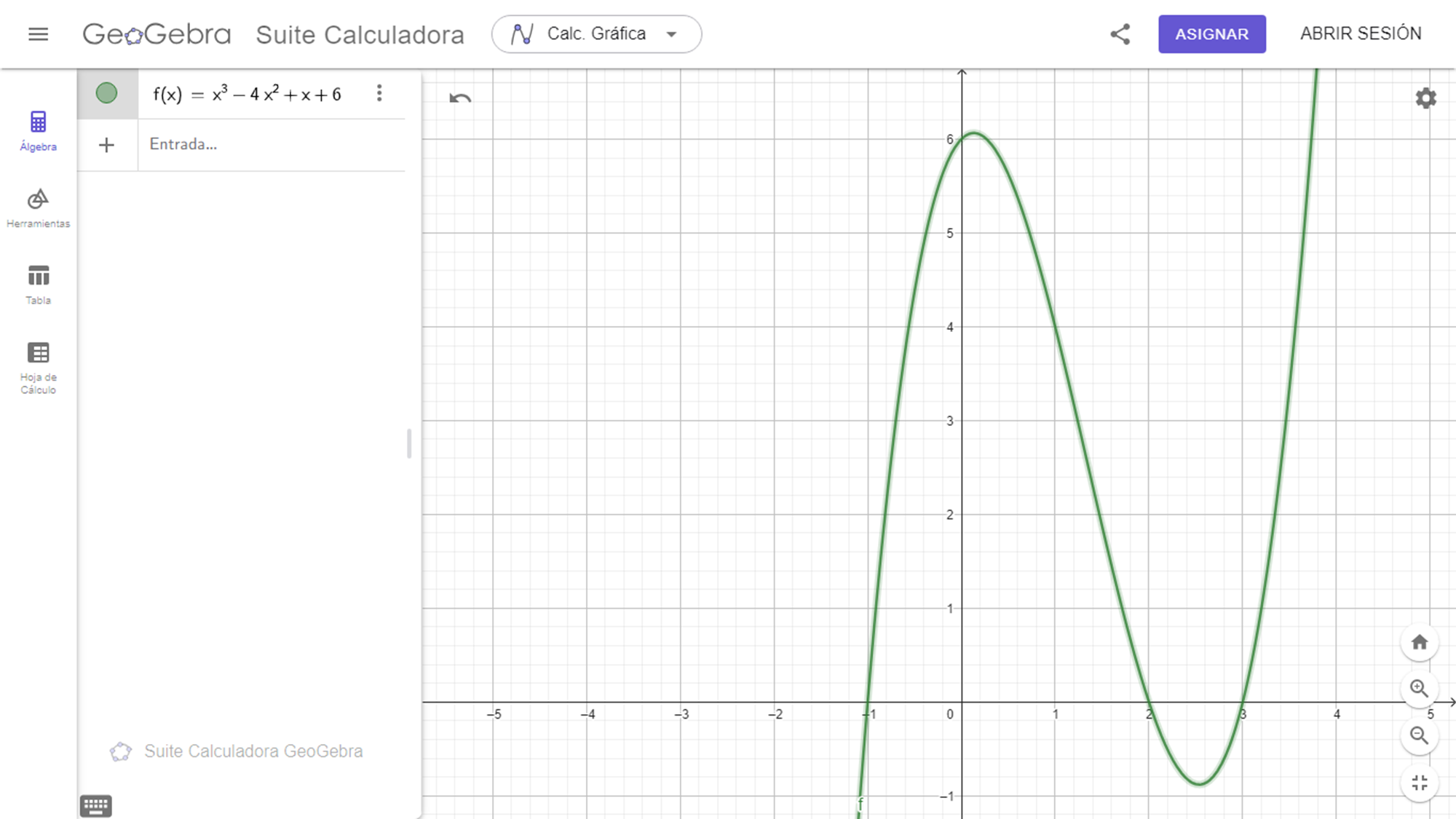
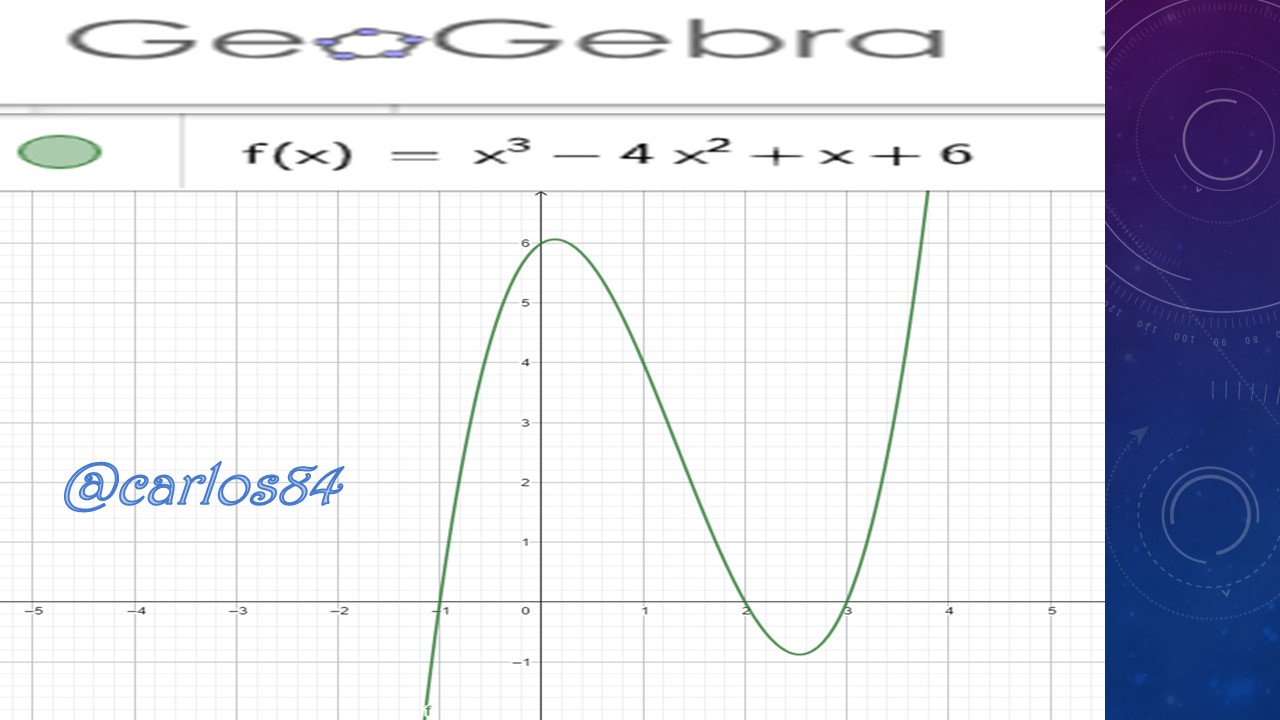
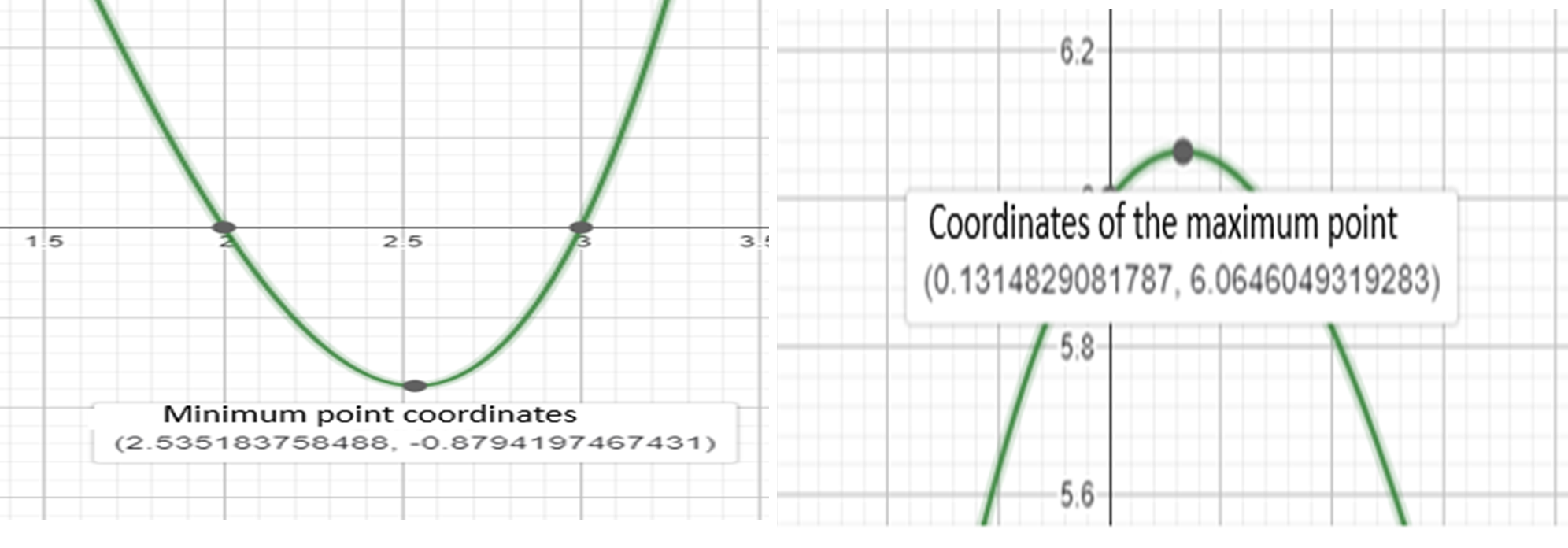
Already with the graph produced by the geogebra program we can see that the cubic function cuts the x-axis at:
X= -1
X=2
X=3
We can also see that the cubic function cuts the y-axis at:
Y= 6.
The coordinates of the maximum point are:
[0.131 ; 6.06]
The coordinates of the minimum point are:
[2.53 ; -0.879]
Is it possible to know the coordinates of the relative extrema of this cubic function by means of another tool?
Of course, which is the purpose of this post, assuming that we do not have at the moment some mathematical software, we can calculate the relative extrema of a cubic function with the criterion of the first derivative.
The criterion of the first derivative what it states is that when we equal the first derivative to zero and we solve the factorization of the polynomial that we have left, the values found are going to be the coordinates in X of the maximum point and the minimum point, therefore we proceed as follows:
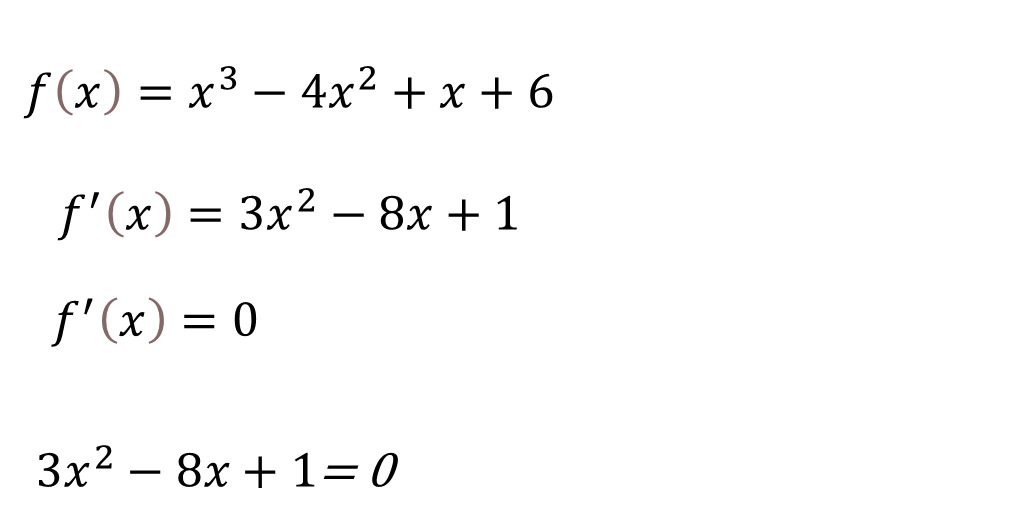
If we find the solution of the second degree equation, we can use the resolvent formula:
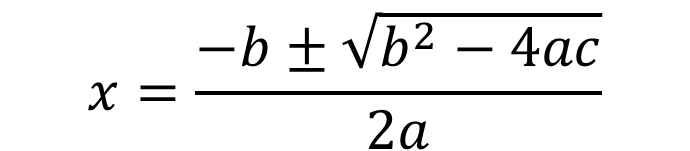
To this end:
a= 3
b= -8
c= 1
Therefore:
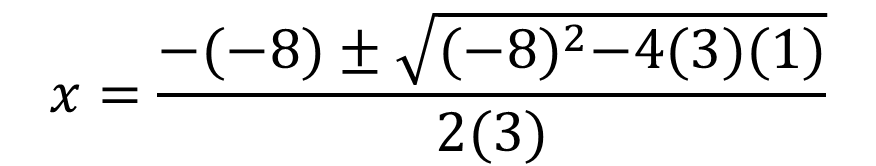
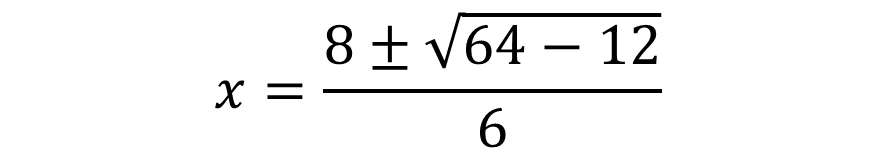
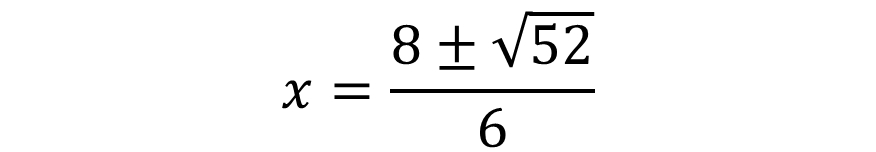

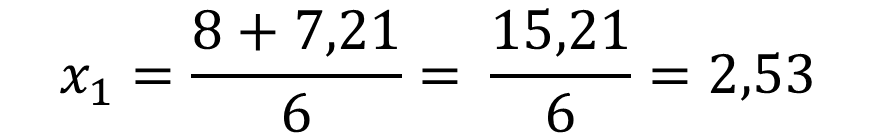
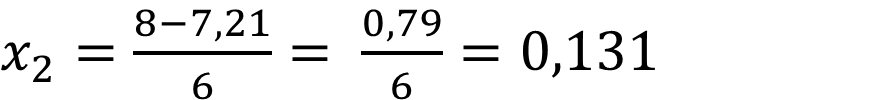
To find the Y-coordinate of the maximum and minimum points, we substitute in the cubic function the value of the X-coordinates of X1= 2.53 and X2= 0.131.
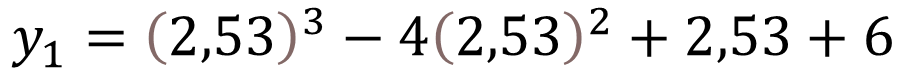
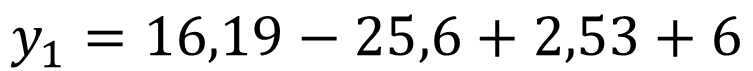
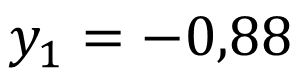
Therefore the coordinates of the minimum point is:
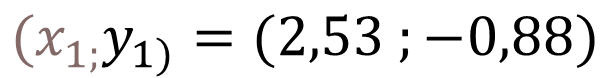
For the maximum point we substitute X= 0.131 in the cubic function:

Therefore the coordinates of the maximum point are:
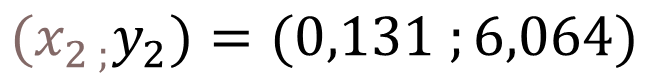
The conclusion to find the relative maxima of a cubic function is that if we do not have a mathematical program or software at hand, we must know that we have the criterion of the first derivative to get the coordinates of the maximum and minimum points of the function.
Recommended bibliographic references
Book of calculus with analytic geometry volume I. Author: Larson and Hostetler. 7th edition. Year 2007.
Note: The images of the cubic function graphs were generated with geogebra software and edited with Microsoft PowerPoint. The equations were elaborated using the equation insertion tool of Microsoft PowerPoint.
Thanks for your contribution to the STEMsocial community. Feel free to join us on discord to get to know the rest of us!
Please consider delegating to the @stemsocial account (85% of the curation rewards are returned).
You may also include @stemsocial as a beneficiary of the rewards of this post to get a stronger support.
Good work but you forgot the importance of checking the second derivative. It has to be non zero for your first derivate zeros to be the max/min of the original function.
They also tell you (if they are non zero) whether your original has a minimum or maximum. If the second derivative is positive it means your first derivative is transfering from negative to positive at its zero and the original function first had a decline in values and after that rises in values which means it's a minimum. Vice versa for the maximum.
Should both derivatives be zero you have a saddle point in your original function.
I will take into account your considerations for a future publication, since the objective of this one was to use the criterion of the first derivative. I will take into account the criterion of the second derivative for my next publication.
Greetings, I hope to continue reading your comments in my next posts.
My comment was trigger by exactly that thought. As I said, it can be misleading if the second is zero as well. In that case you might think you found an extreme while you are actually hitting on a saddle. While at it I added the discussion point about the difference between max and min.
Sorry if that was too exhausting, once I get triggered by math I cannot resist :-P
!BBH
Hello friend, of course I understand you, we are in contact, thanks for encouraging a good debate.
@carlos84! @hannes-stoffel likes your content! so I just sent 1 BBH to your account on behalf of @hannes-stoffel. (2/50)
(html comment removed: )
)