#Ahh...the Möbius Strip

Or, if you are not necessarily mathematically inclined, essentially the recycling symbol:

A Möbius Strip is a surface with one continuous side formed by joining the ends of a rectangular strip after twisting one end through 180°. [source]

So, just like reducing, reusing, and recycling should be one continuous process, the Möbius Strip has one continuous side.

Usually Möbius Strips are the stuff of legend in math texts and introductory Topology Courses for their amazing properties. Some of those properties get pretty crazy with the heavy math involved in the process.

However, some of those properties are pretty easy to access with some paper, scissors, and some curiosity.

If you are so inclined, follow the series of steps along with me! You might get your mind blown in the process.

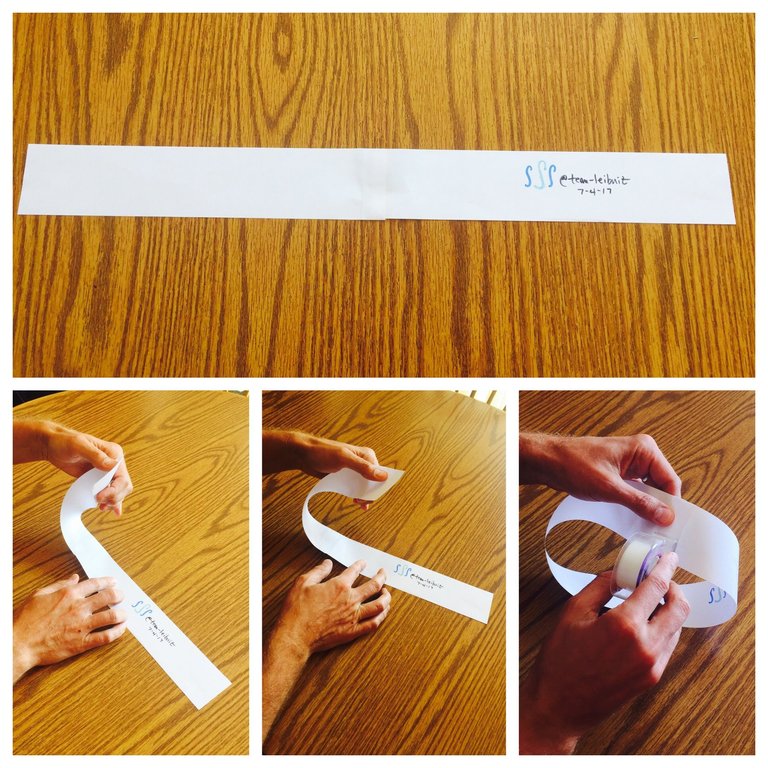
Step 1 - Create the Möbius Strip: The Half-Twist

Cool, you made it! Pretend you are an ant on the surface of this strip. You would keep walking forever and touch every part of its surface. Neat!

Oh, about those "other" interesting properties I mentioned?

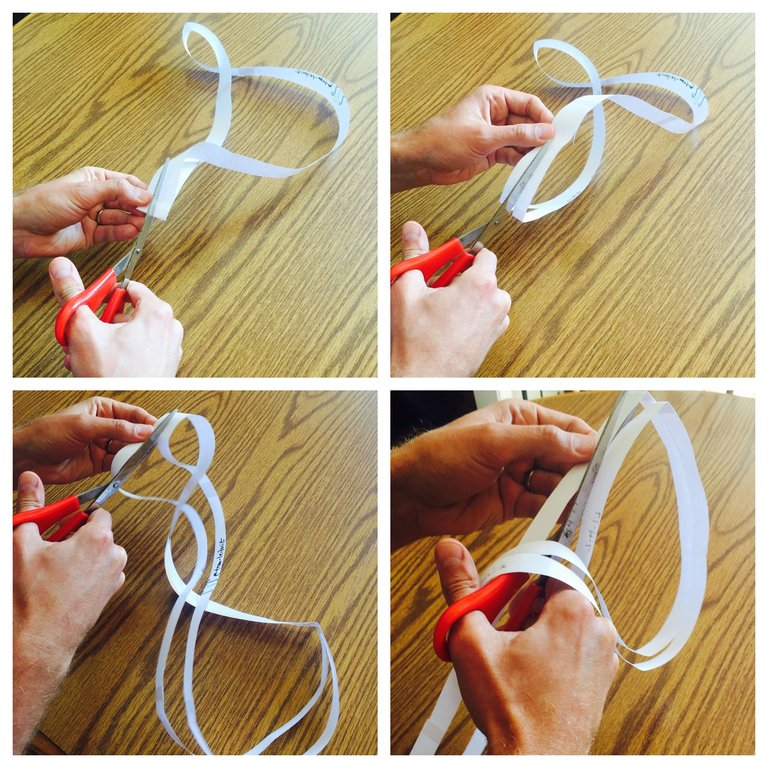
Step 2 - The Cut

What if you cut the Möbius Strip down the center. You would produce two different strips, right? Let's try it out!

Step 3 - The Reveal

This is really quite remarkable.

Our brains might think at first that cutting this shape down the middle would yield two separate strips. Yet, as you can see here, we just get another strip that is now longer in length with an extra loop.

Here is a nice animation of that process in action:

To understand what is going on, you just have to understand the "Topology" of a Möbius strip

When forming the the loop with a 180° twist, you are making a shape that actually has only one side and one edge. There is really no outside or inside to this shape, even though you might think it has one like a traditional loop.

A good way to verify is to take a pencil on the original strip, prior to cutting, and drawing a line along where you are going to cut. You can draw a straight line across the entire shape without your pencil ever leaving the strip! Compare this to a loop with no twist and to accomplish the same feat, you would need to pick your pencil up to separately draw across the inside and outside of the shape.

After you cut the Möbius Strip, try using your pencil to trace around the middle of the shape again. You will see that the loop now has two edges and two sides along with two full twists. It is also twice as long as the original strip.

It is important to realize, its all about the twists!

Without getting super heavy handed into the topology of it all, a good way of understanding cuts on these strips is to think about how they are originally constructed. As long as a loop has an odd number of 180° twists, (180°, 540°, 900, etc.) it will be a Möbius Strip. However, if it has an even number of 180° twists (360°, 720°, 1080°, etc.) it will just be a twisted loop, or more or less a glorified rubber band.

It all means this: If you are to ever cut a twisting non-Möbius loop, you will always end up with two separate loops. However, the twists end up locking the loops together.

Want proof?

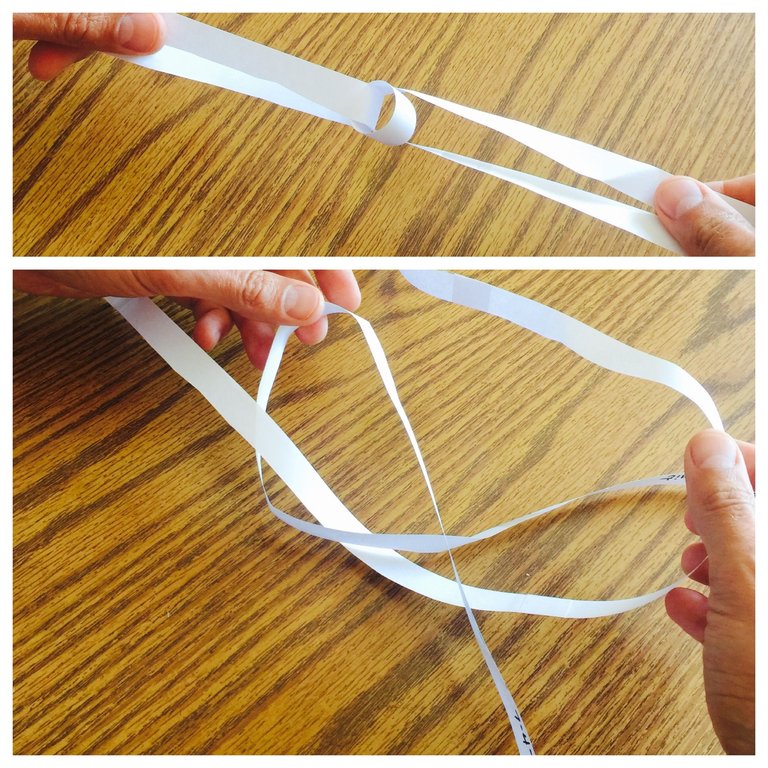
If you recall, when we cut our Möbius Strip in half the first time, it produced a new strip with two twists. If our theory holds, if we are to cut this in half again we should get two separate interlocking loops.

Let's get out the scissors!

Drumroll please....🥁🥁🥁🥁🥁🥁🥁🥁

We now get two interlocked looping strips with two twists each that do not break apart.

Seriously, try pulling on it. They are permanently linked together.

I'm not going to try and cut each again separately, as I think we all get the point. However if we actually did, knowing the pattern of it all by this point, we should expect four small strips with two twists each. Do you think they would still be interlocking? Try it out and let me know what you found in the comments below.

As a matter of fact, you can have even more fun with this experiment!

Instead of twisting 180°, try 540° or even 900°. What happens now when you cut in half?
Try the 180° twist again, but this time cut not exactly in half, but rather one-third of the way from outside. What happens this time?
Try cutting with a 1080° twist. We know we get two interlocking loops, but each with how many twists?

If you come into anything amazing, let us all know in the comments below! Or, just head on over to the mathematics forum over on chain.bb where this post was made. Thanks @jesta for the ability to have such forums!