Fractals is complicated and require a lot of research to get a handle of it.
I first come across fractals and wrote a program to compute at different level of details - precision and each run took a long time - back in 1989. I think the computation will still take a long time unless the computer or the techniques is in line with the fractal mathematics.
In engineering, I learned the power of analog computers and when applied to calculus. I later learned APL - a statistical programming language and marveled at its power and simplicity / conciseness. I just know that there is one or could be developed to facilitate fractals computation and to unleash its power in modeling lifes fractal nature.
You are viewing a single comment's thread from:
Interesting!
What is fascinating is that even though the calculations and the relationship beween the structures are infinitely complex, the rules behind the recursive loop are simplistic.
"The" Mandelbrot set is the set obtained from the quadratic recurrence equation
z_(n+1)=z_n^2+C
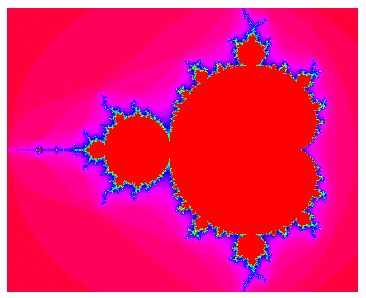
(1)
with z_0=C, where points C in the complex plane for which the orbit of z_n does not tend to infinity are in the set. Setting z_0 equal to any point in the set that is not a periodic point gives the same result. The Mandelbrot set was originally called a mu molecule by Mandelbrot. J. Hubbard and A. Douady proved that the Mandelbrot set is connected.
http://mathworld.wolfram.com/MandelbrotSet.html
Aahhh, the beloved Mandelbrot ;) ... Hi guys... Sorry to hi-jack your thread here (I've bookmarked it for later reading, as I am a crypto trader too).
Thing is, I have Ginabot notifying me whenever anyone says "mandelbrot set", lol... This usually takes me to people who would be interested in the kind of art I do. I use fractal software to create art. Do check it out if your curiosity is piqued =)
@mandelsage
cool! I posted here:
https://steemit.com/artificial-intelligence/@freedomshift/fractals-mandelbrot-set
Thanks for commenting!
Nice art!
What kind of fractal software do you use?
Cool. We studied the Mandelbrot Set and Douady at Uni, brings back memories:)
I remember we played around with setting random parameters to get even more organic depictions.
This is perhaps the most simplistic demonstration of fractals, but, interesting to the general public.
Agreed, the crystal is simple. But it still has some of the fascinating characteristics of more complex ones, like it has an approaching infinite perimeter and the mathematical dimension is not the standard 1D or 2D, but somewhere in between.