Ramanujan's approximation of Pi
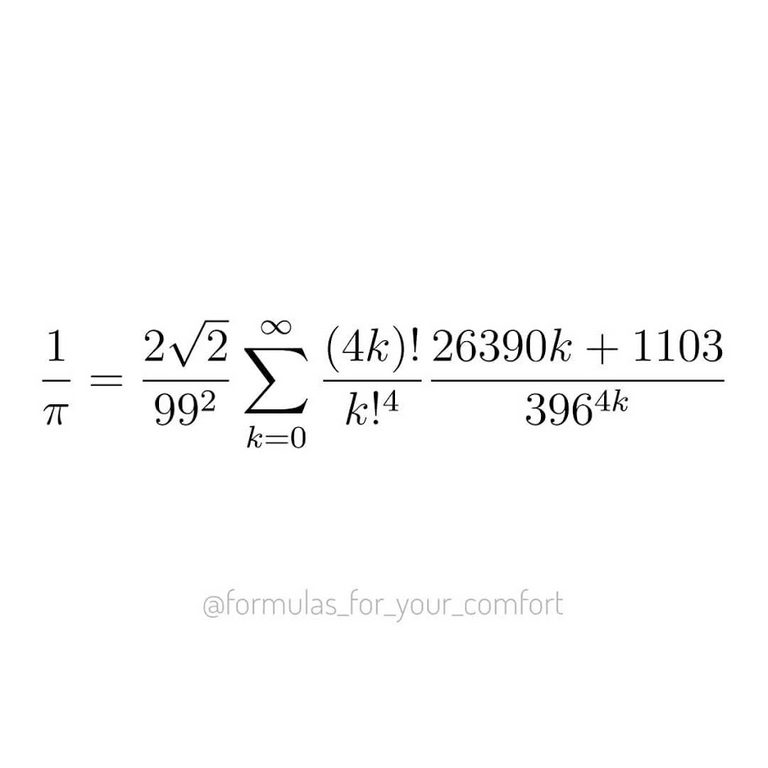
This formula is an expression of pi as an infinite series. It was discovered, purely by intuition (yes, that's possible), by the Indian mathematician Ramanujan. Famous for not proving any of his discoveries, this result isn't an exception: with his godlike intuition, he himself said multiple times that his ideas came directly from god, in his prayers, Ramanujan wrote this result in 1910, in his notebook. More on Ramanujan
This series is rapidly converging, each term of the series adds 8 correct decimals to the approximation of pi. The symbol with k = 0 underneath it and infinity over it is a summation symbol, it means that adding the different values for k = 0, k = 1, k = 2 ... up to infinity will give you 1/pi. Consequently, adding up an infinite amount of terms isn't physically possible, in reality the more terms you add, the closer you'll get to 1/Pi, giving you a greater precision on Pi.
The idea behind the formula is to approach a circle with radius one (with area Pi*1^2 = Pi) with inscribed polygons. The different terms calculate the area of the subsequent polygons (4 sides, a square, then a pentagon, 5 sides, a hexagon 6 sides etc...), a polygon with an infinite amount of edges would therefore be perfectly smooth and since all the different polygons are inscribed in a circle, an infinite sided polygon is actually a circle and its area is therefore Pi.
This formulas doesn't actually calculate the area of the different polygons, but that's the idea behind it, it's part of a family of infinite series which rely on that idea : the Ramanujan–Sato series
Never the less, this formula is unique because of the way it was found, through pure genius and imagination.
...
formulas_for_your_comfort on Instagram.
If you'd like to learn about different formulas and their background, don't hesitate to follow @formulas or check out
Any advice is welcomed, just starting out on steemit ! Thanks for making it to the end !
This post has been voted on by the SteemSTEM curation team and voting trail in collaboration with @curie.
If you appreciate the work we are doing then consider voting both projects for witness by selecting stem.witness and curie!
For additional information please join us on the SteemSTEM discord and to get to know the rest of the community!
Hello @formulas! This is a friendly reminder that you have 3000 Partiko Points unclaimed in your Partiko account!
Partiko is a fast and beautiful mobile app for Steem, and it’s the most popular Steem mobile app out there! Download Partiko using the link below and login using SteemConnect to claim your 3000 Partiko points! You can easily convert them into Steem token!
https://partiko.app/referral/partiko
Congratulations @formulas! You have completed the following achievement on the Steem blockchain and have been rewarded with new badge(s) :
Click here to view your Board
If you no longer want to receive notifications, reply to this comment with the word
STOP