
So far, we only know of one example where a planet has given birth to some form of life. And given this there doesn't seem to be much hope to learn of the Universe’s fertility for such life. Well, what is the probability of us being alone?
Often we associate being “alone” as being alone right now. Asking this question will always result in a very small probability, in fact it will always be zero. The probability of anything at a single point in time will always be zero, because when we take the integral of any probability function over the interval [a, b] where a=b the area over that interval (i.e. the probability) is zero.
Frank and Sullivan asked exactly this question which they call the ‘‘cosmic archaeological question’’. How often in the history of the Universe has evolution ever led to a technological species, short or long-lived? They modified the famous Drake equation to address this question, because the Drake equation calculates how many technological species exist now. When the Drake equation was written, observations didn’t provide accepted or well determined values. Fortunately for Frank and Sullivan, they did.
Their equation is simple:
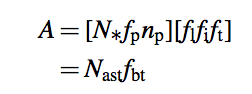
The parameters are:
• A - the total number of technological species to have formed over the history of the observable Universe
• N* - the total number of stars
• f_p - the fraction of those stars that form planets
• n_p - the average number of planets in the habitable zone of a star with planets
• f_l - the probability that a habitable zone planet develops life
• f_i - the probability that a planet with life develops intelligence
• f_t - the probability that a planet with intelligent life develops technology like our own
They asked “Has even one technological species ever existed in the observable Universe?” Using N*=2x1022 for the total number of stars and ‘A’ was set to a conservative value, 0.01. This means that if we were to run the universe 100 times then the we would only occur once. The answer they arrived at was 2.5x10-24. They went on to add an additional parameter, Rs, that generalizes this result for areas within the universe. For example, our own galaxy would have an f_bt value of 1.7x10-11.
But what does this mean?
Well, an f_bt value of 1.7x10-11 means that if we were to rerun the universe 1700000000000 times, in only one of those reruns would there be one or less technological species. Using their model we can say with some confidence that another technological species has likely occurred in the Milky Way.
In perspective, the complement of the original question - “Has more than one technological species ever existed in the Milky Way?” results in a 99.999999998% chance that another technological species has occurred in our home galaxy. Even if only one in every one hundred million stars has a technologically advanced species today, there would be total of about 3,000 such civilizations in the Milky Way alone.
But, one thing that Frank and Sullivan did leave as a moot point was the lifespan of a civilization. Even if there are 3,000 civilizations in the Milky Way given the extremely long life of the universe and the short life spans of civilizations it is unlikely that two would ever come about at the same time.
Do you think we’re alone?
Frank and Sullivan Paper - http://online.liebertpub.com/doi/pdf/10.1089/ast.2015.1418
[Image Source: pixabay.com, licensed under CC0, Public Domain]
Simple argument. The law of large numbers...
Since we exist, a probability for life exists. If the universe is infinite, then life has to occur an infinite amount of times.
This is a good point - indeed the probability of anything over an infinite time interval would be 1. However, the universe might not be infinite and Frank & Sullivan only assumed the probability over the observable universe, ~47 billion lightyears, a very large number! Yet, still infinitely far from infinity.