Fundamentals of Filtration
In the engineering world, an important aspect of any processing site is Filtration. Filtration is the separation of solids from a liquid by the passing of a suspension through that of a pereable medium that thus will retain the solid particles.
Filtration is a process that does not occur naturally but instead needs a driving force to promote the process. The driving force of filtration, as mentioned in the previous post, is referred to as a pressure drop. A pressure drop is an overall decrease of pressure across a systems’ medium and can be achieved in one of the following ways:
- Gravity
- Vacuum
- Pressure
- Centrifugal
- Diffusion
- Inertial
By using the aforementioned driving forces, 2 types of filtration methods can be distinguished in industry and those are:
Surface Filtration
Deep Bed Filtration
Surface filtration is conducted by the use of surface filters and are used for a latter branch of surface filtration, known as Cake Filtration. During this form of filtration, the solid particles that are prevented from filtering through are packed together above the membrane medium. Due to the accumulation of layers of these non-filtered solids, an amalgamated layer is formed. This layer is referred to as a cake. This form of filtration only takes place on the upstream side of the membrane. Since this form of filtration occurs in a vertical direction, it can be identified that the driving force behind this form of filtration is that of Gravity. The filter medium of cake filtration has a low pressure drop across the medium itself. It can be noted, that since large particles in the sequence do not get filtered and forms the many layers of the cake, these particles themselves become a filtrate medium too as the fluid flowing through encounters these particles as well.
For the method of Deep Bed Filtration, depth filters are employed. This branch of filtration is when particle deposition takes place within the medium itself. Due to this occurring, we must note that the cake will therefore form internally within the medium. Due to this internal formation, the cake will be contaminated by impurities to such an extensive degree that it would not be economical to try to improve the purity of the cake. For this reason, we regard the cake produced via deep filtration to be an undesirable deposition. The reason the cake occurs internally is because these large particles are smaller than that of the openings on the surface, thus these particles filter through. Once internal, these particles are now captured by pores on the inside of the medium. Gravity, Inertial or even Diffusion are mechanisms that allow this form of filtration to be possible.
How to calculate the overall pressure drop:
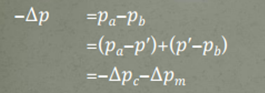
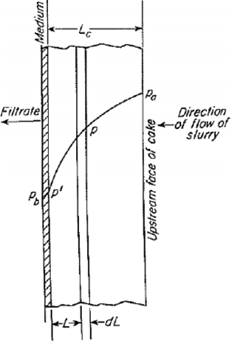
-dP = overall pressure drop
P(a) = pressure at inlet
P(b) = pressure at outlet
P’ = pressure at the boundary between the cake and the boundary
dP( c ) = pressure drop occurring along the cake
dP( m ) = pressure drop occurring across the medium
Pressure drop through a filter cake:
To understand the concept of a pressure drop across a cake filtration process, we must first understand this fundamental concept:
When the cake filtration (batch)is initiated, the entire pressure drop that is available to the process at that specific time (t=0) is across the medium itself only. This is because, when the process starts, there is no cake that is available at the time, cake only becomes available after a certain time period (t=t(1)).
Due to the pores in the system (medium) being generally small, with the addition of typically low flow rate of the filtrate, Laminar Flow conditions are observed. As per my previous post, The Hagen-Poiseuille Equation (explained in great detail) can be used,
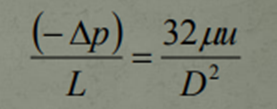
If filtration is to occur through a packed bed, such as one in a Fixed Bed Reactor, the Carman-Kozeny Equation (also explained in great detail in my previous post) can be used,

Where:
k(1) is a constant that changes per the packing arrangements.
For a set of randomly packed particles that have the physical characteristics of a definite shape and size, k(1) is 4.167.
It should also be noted that the pressure drop obtained can often be expressed as a function of other variables, which in the case of filtration, is often a ratio of surface area to volume ratio of the particle, rather than directly using the particle size. This will therefore cause the Carman-Kozeny Equation to become,
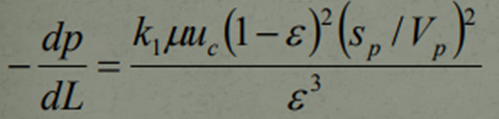
Specific Cake Resistance:
The cake is formed during filtration has individual surfaces that may promote or demote filtration. In most cases, these surfaces perform negative work as it mainly offers resistance to filtration. Therefore, Specific Cake Resistance is a measure of the degree of resistance to flow for filtration of the cake. It is often expressed by a single equation consisting of variables that have individually specific values per material of cake,

Filter Medium Resistance:
Similarly, to that of cake resistance, Filter Medium Resistance is a measure of resistance produced by the medium itself when filtrate flows through. It should be noted that Filter Medium Resistance is analogous to that of Specific Cake Resistance and can be expressed as,
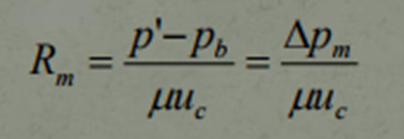
The overall pressure drop will now change because it happens independently across the medium. The pressure drop across the filter medium can therefore be expressed by the following equation,
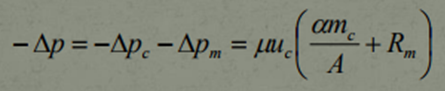
It can be noted that when the cake formed consists of a substantial thickness, the pressure drop across the medium is small in comparison to that of the pressure drop across that of the cake. When this occurrence is noted, we may ignore the magnitude of the Specific Cake Resistance can be ignored.
Working with these large equations can often be time consuming and may lead to errors in calculations, therefore to prevent these errors from occurring, certain variables are written as functions of other variables. Upon derivations and methods of conversions to produce functions, the following equations were produced:
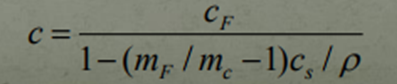
Where,
C = particle mass deposited per volume of the filtrate
C(f) = concentration of solids in the filtrate
M(f/c) = mass of the wet cake and dry cake respectively
C(s) = concentration of solids in the slurry mixture
The material balance across a system is often referred to as the Filtration Equation. The derivation from first principles produces the following equation,
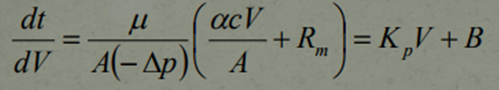
Where K( p) and B represent:

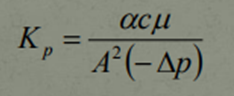
When filtration is occurring at a constant pressure, the Filtration Equation can be reduced to the following equation,
NOTE: this equation is only stand for when pressure is constant and no other variable i.e. not volume or time
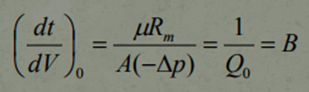
This brings us to the end of Filtration Fundamentals. I hope that you know have a better understanding in the world of engineering pipework and what an intricate job it can be. Stay tuned to my blog for more on filtration in my future posts.
Images are linked to their sources in their description
The End
References:
[1]http://www.filtration-and-separation.com/cr_filtration/sld018.htm
[2]http://en.wikipedia.org/wiki/Filtration
[3]https://www.lenntech.com/chemistry/filtration.htm
[4]https://en.wikipedia.org/wiki/Filtration#Methods
[5]https://ocw.mit.edu/courses/chemical-engineering/10-445...for.../lecture_9.pdf
Being A SteemStem Member
A filter cake? I couldn't prevent myself from thinking about the eatable cake :D
Haha if only making edible cake was as fun as science :'D
It is funny as well! A different fun, however :)
So humorous, trust me, I love eatable cakes than you do #smiles
How do you know how I love then? :D
The implications and applications of pressure drop in engineering and science is invaluable.
Thanks for this, I surely learnt something. It is nice being on your blog. I am a fan and writer in the stem field too - a student though.
Nice having you here to guide.
In processing, it can considered the single most important process to understand. I am more glad that you have learnt from this. It makes my deed of teaching all the more enjoyable.