Image Processing - Stacking

Active Galaxy Centaurus A imaged by the author. To obtain this image 1370 exposures of 4 seconds were stacked using the summation method
In the previous part of this series (part 8), I examined a typical astronomical image processing workflow as shown in the following diagram.
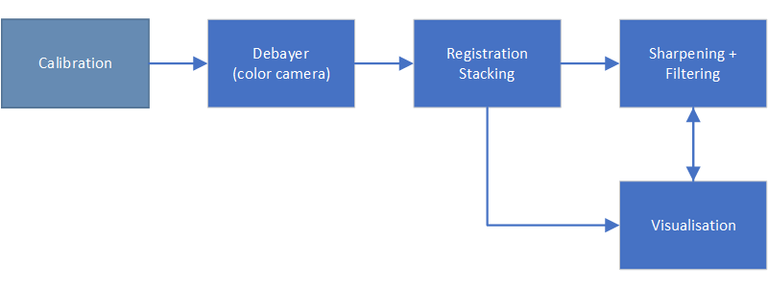
Now we shall look at stacking (step 3 in the workflow), as well a bit of background on why it works (and why astronomers love image stacking!).
Intro
Image Stacking is the technique of creating a single composite image from multiple photos of a subject. There are several common methods of stacking which we look at in this article. At the end of this blog I will talk more in depth with some theory, but the next section is more the practical application.
Stacking Methods
Summation and Averaging
The summation method creates a composite image using addition to combine the images. In Photoshop or Gimp, this would be equivalent to placing the images on separate layers and setting the layer mode to addition.
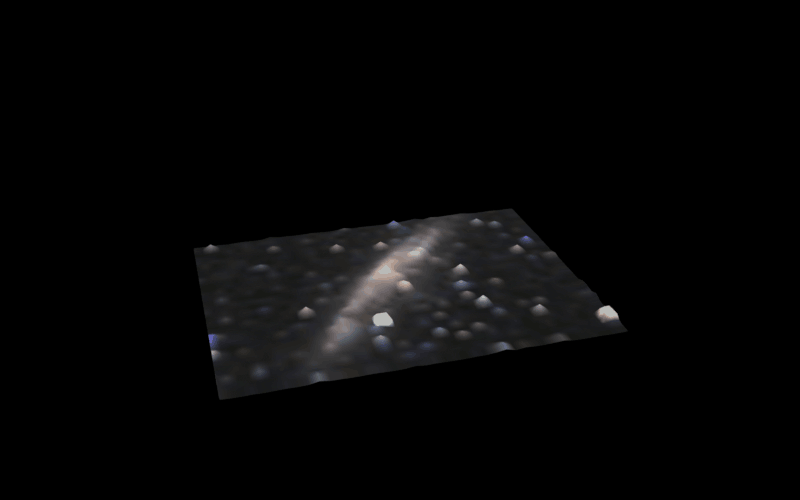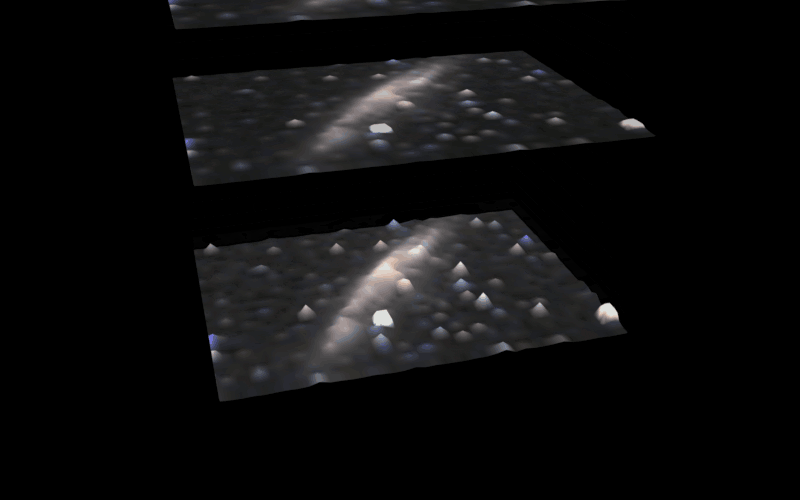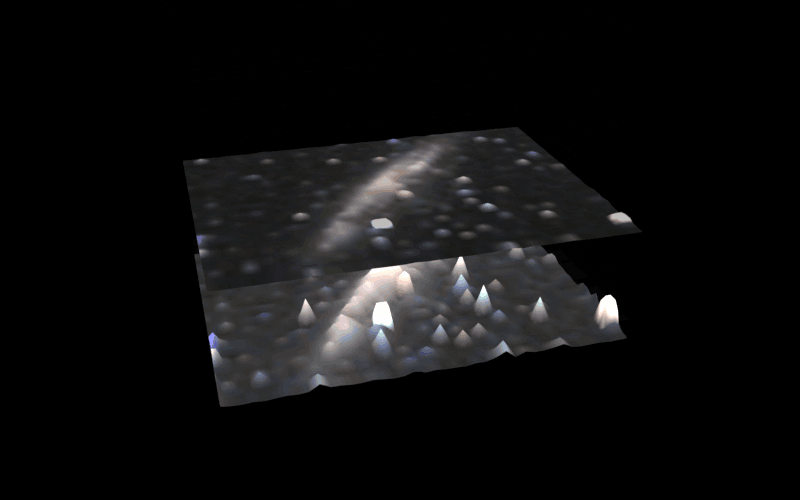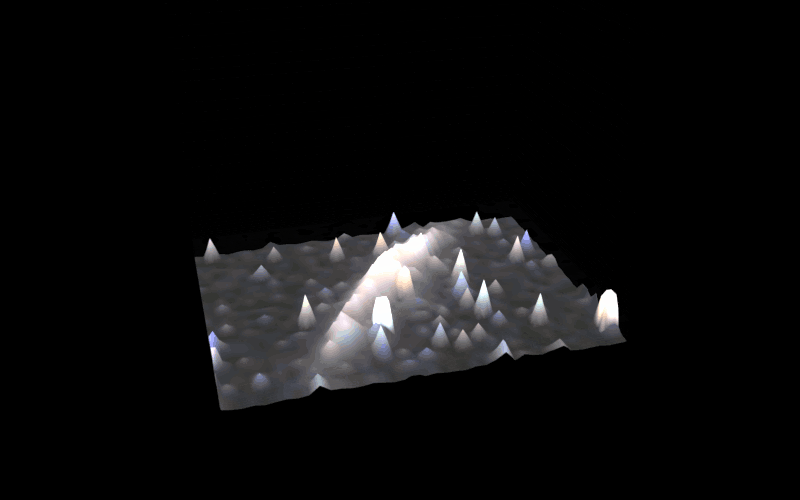
Summation method of stacking, showing the stacking of 4 images. The image has been displaced topographically proportional to pixel brightness
This method is very fast but it's Achilles heel is if one the images have a flaw this will be visible in the final stacked image. This flaw could be anything from an aircraft flying through the frame to a cosmic ray strike on the sensor.
Example : Here is an example of stacking a sequence of 16 individual photos of galaxy NGC4945. Here is a screen capture of the thumbnails for each of the photos. Note that the last photo 'Capture_0016.fit' has an aircraft trail through the bottom left and we will see how we can deal with this.

This is the image list thumbnails used to generate the example stacked image (see below)
For reference here is what a single image looks like. You can see the background is quite noisy.

Single photo 'Capture_0001.fits' from above Sequence shown full size
When the 16 photos above are stacked using the summation method the following image is the result. Note the noise is much less and a lot more detail is now present. However, note that the aircraft trail from photo Capture_0016.fits unfortunately still shows in the stacked image. This is the major downside to this method of stacking.

Summation stacking of 16 images. Notice how much better the image is compared to the single frame above, although unfortunately the aircraft trail present in image number 16 is clearly visible
Median
If a median method is used instead of summation, the rejection of flaws in a single image is greatly improved. The median method takes the statistical median value of the images to obtain the stacked image.
The following image is a median stack using the same image set as the one used for the previous image. Notice that the trail is almost gone (only a faint trace can be seen). The median stacking method is much slower than summation and it may be just as effective to remove the flawed image from the image stack if the number of photos is large.

Median stacking of 16 images
Sigma Rejection
The Sigma Rejection method of stacking removes any pixel values in the photo stack that are very different in brightness from the other pixels. It does this by using the standard deviation of the pixel values, and removing any values too far from the mean.
Sigma Rejection is best at removing single frame flaws in an image stack, as can be seen in the following example where the 16 photos have been combined using this method. Note the aircraft trail is now completely invisible.

Sigma Rejection stacking of 16 images
Theory and Background
Image
An image is represented in a computer by an array or matrix of pixels as shown in the following diagram. Each pixel in the array is referenced by specifing the row and column position. The pixel value represents the brightness of the image at that point. For color images the array pixels will also contain the brightness for red, green and blue which is stored in memory as a single 24,48 or 96 bit value.
![]()
A typical image can be considered to be an array or matrix of brightness values as shown here. Pixels are referenced by a column and row index number
Summation Stacking
The simplest form of image stacking is summation where the pixel values at each pixel position (specified by row and column) are added together across all of the images. In mathematically terms the operation can be described as follows:
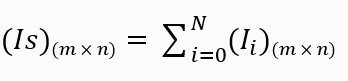
Where IS is the stacked image array and Ii is a sequence of N image arrays to be added. m x n refers to an image array of width x height
Signal to Noise Ratio (SNR)
When images are summed together correlated information such as stars and galaxies will generate a signal in direct proportion to the exposure time. Therefore by doubling an exposure all the stars in the image will register twice the signal. By correlated, we refer to any signal source that is persistent across all the images in a stack.

Where S is the total signal of an object, N is the number of the exposures and S0 is the signal of an object in a single exposure
In real images, random noise is almost always present. Even if the camera is perfect and introduces no noise of its own, light itself generates noise (called shot noise) and this is not correlated from one image to the next. One property of this noise is that it does not add proportionally in stacking. In fact, noise sources combine together as follows:
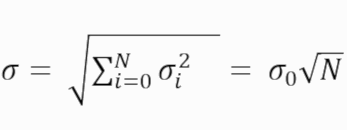
Where σ is the total noise in the image stack, N is the number of the exposures and σ0 is the noise in a single exposure
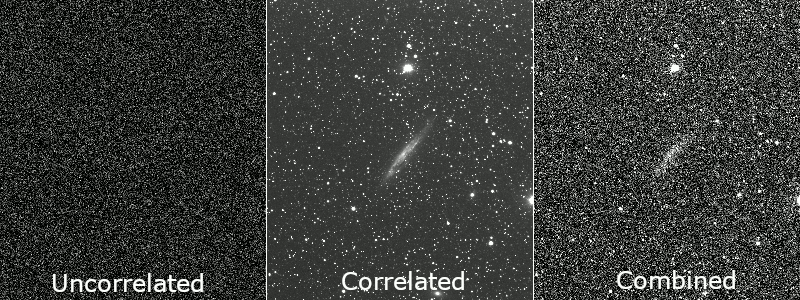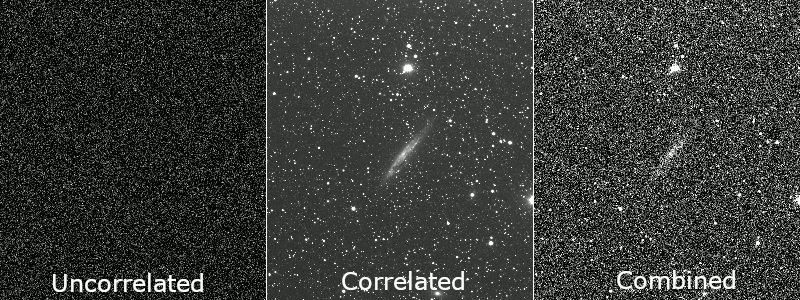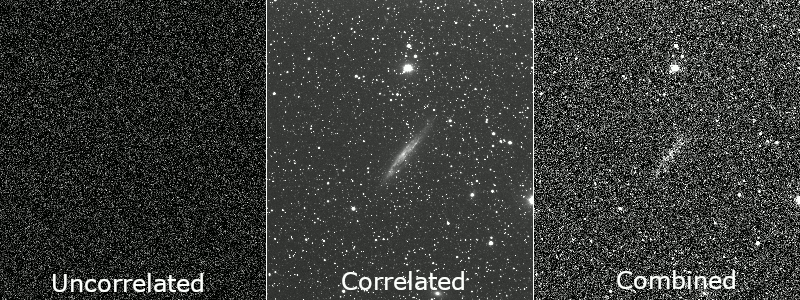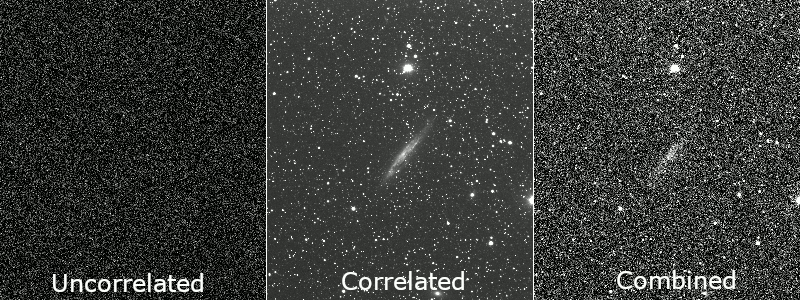
Real images contain random noise (uncorrelated information) and detail (correlated information). When you combine them you get the real image on the right
Once we know the signal and once we know the noise level, we can compute Signal to Noise (SNR) as just S / σ. Typically, for basic detection SNR > 3 and for highly confident detection SNR > 5. If we just wish to compute the improvement in SNR due to stacking we can calculate it as follows:
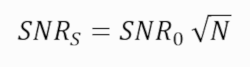
Where SNRs is the SNR of the image stack, N is the number of the exposures and SNRσ0 is the SNR of a single exposure
Hence in the image in title, 1370 images were added in the stack. This should have resulted in an improvement of 37 times in image quality over a single image. Now you know why astronomers love image stacking!
Why can't I stack the same Image?
Some people have asked why can't one just stack the same image over and over again. The reason is all the detail and noise in the image is correlated between the images, so there is no net gain in SNR.
Observations versus theory
It is interesting to see that those above predictions work very well in practice. We can actually measure this. The following data shows actually measurements that were made off the photos made in the earlier examples.

Actual measurements (point markers) versus Theory (solid line). Observation is indeed consistent with predictions!
Conclusions
In this article, we looked at a couple of different methods for image stacking (as well as some theory). We saw how effective stacking was in improving image quality. Next time around I will be looking at filters with a few examples to show you just how effective they can be at improving images.
References
- Buil, Christian. CCD Astronomy: Construction and Use of an Astronomical CCD Camera. Richmond, VA: Willmann-Bell, 1995.
NOTE: All images in this article are the author's, please credit @terrylovejoy if you wish to use any of them.


That summation method of stacking gif fits its name
That's good to hear!
It's impossible not to give you the upvote. It's a perfect post:
mean vs median vs "the value in the galaxy far, far away"
SNR - explained
Actually... Something could be done... Yes it sounds like the mathematical black magic but check the references from Ivica Kopriva. He was working a lot on under-determined blind source separation problems and even succeed to extract multiple components from the single image with and without the extension of dimensionality.
Thank you very much @alexs1320 I will definitely check out Ivica Kopriva's work. However, It does sound like it is more a technique of extracting more out of a single image.
We had tried something for FTIR images, and after extracting the components with the meaning, there is a lot of nice noise to be discarded and some background artefacts. The problem was that it looked a bit ugly (a bit artificial) because there were completely noise-free parts and then the areas with information and some noise. Maybe it will be nicer with your kind of images.
Interesting one @terrylovejoy
The gif showing stacking reminds me of Atomic Force Microscopy used to image the structures in nano range.
I am trying to avoid the "nice post!" comment but that's what came to my mind.
First time i am hearing stuff like this. And it was really worth the read.
The mentor gif is cool too!
Thank you @rharphelle ! I am happy with "nice post" btw :)
Great work Terry :-)
Thank you Kevin!
Atmospheric distortion makes the star positions waver a lot (twinkling or scintillation).
There should be times where the atmosphere calms down (very briefly) and you get a good clean undistorted image.
Do you ever then just use those few frames where the stars seem to be in their correct (or nearly correct) and undistorted positions and then just add those up?
I imagine that you would have to throw away a lot of frames to make this work but you would get a cleaner image at the end?
There are rare times the atmosphere settles down. What usually happens with planetary imaging is that perhaps 50% of image frames are used in the final image. With deep sky images generally, you must use longer exposures so the images are always slightly blurred and so you use most of them.
With the next generation or 2 of camera sensor's, it may be possible to nearly eliminate read noise so we will be able to use very short exposures. Already there are such devices that called EMCCD but they are very expensive.
Great post. UP4YOU :)
@michalx2008x . Thank you..btw what is "UP4YOU" ?
Upvotes :)
Thank you! This is a very informative and thorough post. Learned something, and will explore some astro photography soon, will be using those methods on night Street photography too.
Will be interesting to see how you get on. You might get some interesting effects for normal photography.
What do you mean when you say
exposure time, Is this the time that refers to the waiting time during the image capture? Or is this related to the number of photons captured per unit time?Interesting read by the way.
Hi @sinbad989 exposure time is the total time the camera's shutter was open taking the photo/s. Thank you for stopping by and commenting :)
wow. This is one of the secrets behind super clear pics of galaxy and stars. I though you people are using super high tech camera equipment to get clean images. Thanks for explaining this in a scientific manner.
Cheers man.
The camera helps a little, but even the cameras we are using have sensors that are being used in normal digital cameras. The camera used for the example in this article has the same sensor as the Sony RX100.
nice. This is really high quality processing of those images then.
Can I think this also like increasing the volume of an audio file, where the noise also gets equally amplified?
Yes that is a perfect analogy. In fact it is more than that, it is subject to the same principles.
Great post as always ...maybe you can change the title to ‘Intermediate’ because this series is too good for ‘Basic’ ;)
I thought about that, but the stacking is essential for good astrophotography at least for amateurs. But then you don't have to necessarily understand any of the theory to use it.
I have come across image stacking a couple of times but never really looked keenly into it, this very much enlightening.
Congratulations @terrylovejoy! You have completed some achievement on Steemit and have been rewarded with new badge(s) :
Click on any badge to view your own Board of Honor on SteemitBoard.
For more information about SteemitBoard, click here
If you no longer want to receive notifications, reply to this comment with the word
STOP