English
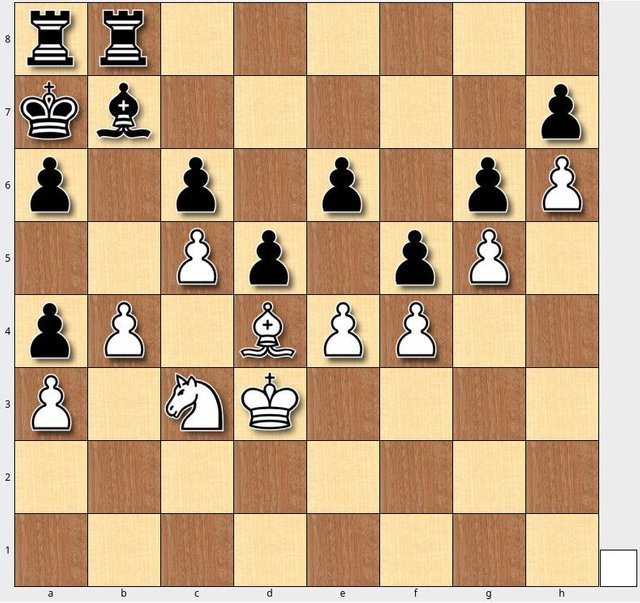
I just created the chess problem below for you to show you the limits of current chess software. Yes, chess programs beat the best chess players in tournament games, but that doesn't mean they have no weaknesses.
To solve this chess problem I invite you explicitly to use any optional chess programs beside of your own brain. :) This is a position where human intelligence should still be superior compared with the calculating power of computers ... we will see ...
Anyway the aim is not to lose with the white pieces in the position you see below. How can white save himself in this seemingly hopeless situation?
Deutsch
Ich habe mir gerade für euch das unten dargestellte Schachproblem als ein Beispiel dafür ausgedacht, wo derzeitige Schachsoftware an ihre Grenzen stößt. Ja, Schachprogramme besiegen die besten Schachspieler in Turnierpartien, aber das heißt nicht, sie hätten keine Schwächen.
Um dieses Schachproblem zu lösen, lade ich euch ganz ausdrücklich dazu ein, neben euerm Gehirn beliebige Schachprogramme zu Rate zu ziehen. :) Hier handelt es sich um eine Stellung, in der menschliche Intelligenz noch bessere Ergebnisse als maschinelle Rechenpower erzielen sollte ... wir werden sehen ...
Jedenfalls ist es das Ziel für Weiß, in der Position unten nicht zu verlieren. Wie kann Weiß sich in dieser hoffnungslos erscheinenden Position retten?
Jaki you are sooo pleasant. Thank's for the prolongation
After two trials, now I take my third chance. Others not seen to play chess and our champion @freiheit50 seems to be also busy in another matter. Take my third strike (no computers aid needed): c3-b5!, a6xb5 (c6xb5 is obsolete), e4-e5 and neither white nor black is able to win the game. So easy…
Congratulations!
Yes, white has to play 1. Nb5+! . Black has now only two possible moves: After 1. ... cxb5? he will be checkmated by 2. c6# (check of the bishop). Therefore the only possibility is: 1. Nb5+ axb5. But then white plays 2. e5 and the position is blocked forever. As the black bishop is moving on the light squares the own pawns are like an impenetrable fence. White only need to wait and move its king around.
Why computers cannot find this solution? Because there are still many possible black moves. They cannot recognize though that there is no chance (and also will not come in future) to break through the pawn chain. In this position it is important to recognize an unchangeable structure on the board instead to calculate very fast.
Great blocking strategy! I guess you got inspired by the famous chess problem of Rudolph, W., where 1. Ba4+! saves the draw:
Yes, indeed!
And the computer will never end the game as drawn? That's not good.
The game will end because of the "fifty-move rule":
The chess software will recognize it cannot win some moves before the fifty-move limit is reached.
As I said, normally chess programs play great chess ... This chess problem was designed to point out an amazing weakness of current chess software (as you proved yourself humans are much better in this special kind of positions). https://en.wikipedia.org/wiki/Fifty-move_rule
Did you fiddle out this funny disfunction or is it a common knowledge of chess professionals?
This special weakness of chess software is well known by insiders. But the position I gave you was created by myself (I managed to combine the feature of a blocked position with a tactical sacrifice of the knight on a square where it can be taken by two different pawns).
The problem in a real game is that the human player very rarely reaches these kind of positions which the software doesn't understand.
I did explain above why the engines cannot find it. They don't recognize long lasting structures and patterns. They are unbeatable when they have to find long forced tactical variations but here after the second white move black can still long time shuffle around its pieces and try many maneuvers, but all that doesn't change the fact of the impenetrable pawn chain.
all current rating lists of chess programs.
But of course I cannot guarantee that there is no single engine which could solve the problem (there are many hundred chess programs available nowadays). I do have in mind two engines with detectors of blocked positions: you could try "Crafty" and / or "Shredder" and lets see if they may solve the problem. I tried the strongest available chess engine (Stockfish, https://stockfishchess.org/), which is leading
Very interesting problem! Thanks for sharing! Unfortunately I didn't find time to think about it til now.
I cannot decide e4xd5 or e4–e5. One of them. But now I'm in a risky mood
Why? Because the totaly blocking of your way down to the white area, was an idea but it doesn't work anyway. When I do e4-e5, you will open the gate on the a-line.
No, my friend ... if you open the position, finally the black rooks will enter your area, so that black can win!
Thank you, Jaki. I thought the machine will answer e6xd5 and then c3xd5 I collect a knight. But you are right. After this „win“ the machine wouldn't do c6xd5 to run into checkmate. Now two highways are open for the black distance warriors. Yes, it's a small chance to close all roads down to white.
The problem is that after 1. e5 the position is still NOT blocked, because black has the option to push a6-a5 sooner or later. No, also after 1. e5 black will still win!
By the way: I didn't say the machine necessarily plays with black: This is an artificial position which I "created"/"invented" to show that chess programs are not able to find the way to draw with the white pieces. Humans like you should be able to find it by logical thinking. :)
Exhellent job ..!!
Up now :)
Thanks! :)
I will be away from computer for a while now. Of course I will give you the solution later in case nobody should find it.
Lieber Jaki, ich habe, genau wie Du, ein Zeitproblem. Mach mal langsam mit der Auflösung, bitte. 15 Minuten sind jetzt schon arg eng!
Oh, ok - I thought you gave up already. :-)
Ok, if you still want to try I will extend the remaining time to solve it! :)