Cite your source, please. That graph conflicts with the following, which claims that the overlap between the two distributions is small:
"The least intelligent 10% of Whites have IQs below 80 (low functioning) while 40% of Blacks fall below this level.
"Only one Black in six is more intelligent than the average White; five Whites out of six are more intelligent than the average Black.
"About 2.3% of Whites have an IQ of at least 130 (gifted), 20 times greater than the percentage of Blacks who do; only 0.00044% of African Blacks have an IQ over 130. 80% of gifted American Blacks have White admixture.
"Geniuses by Race (IQ 140 or higher):
• African Blacks 1:3,500,000 (0.000003%)
• American Blacks 1:218,000 (0.0004%)
• Whites 1:83 (1.2%)
"The per capita genius rate for Whites is 41,000 times higher than it is for African Blacks. If all Whites in America were replaced by Blacks, the number of geniuses in the country would fall from about 2.4 million to only about 1,000."
The above is clipped from the Radical Capitalist article, which cites the refereed article. I have not yet read the refereed article, which is 60 pages long, but plan to do so to verify that the other article is citing honestly.
But my question remains, WHAT IF? Rather than argue whether the difference exists, we should first confront what the implications would be if it is true and whether we are capable of admitting that it might be true. If we cannot admit that it might be true, then we cannot hope to be able to reason impartially about whether it is in fact true.
Rather than argue whether the difference exists, we should first confront what the implications would be if it is true and whether we are capable of admitting that it might be true
ya think? When has the truth ever mattered?
Hey, give me the benefit of doubt! Remember, the standard deviation for whites is higher, which is why there are so many morons like me who can't understand your cryptic posts. :P
Ok. For the normal probability density function, it is the parameter that "means" the amount of variation around the mean. (See the sigma in the formula for a normal PDF here: https://en.wikipedia.org/wiki/Normal_distribution)
But I don't know what you are getting at? And it's probably going to take me a few days to find time to read the refereed article.
You edited your post to add the diagram and the second question after I responded to it. The most useful answer to your first question is that any normally distributed random variable has a probability of approximately 95% of being within two standard deviations of the mean (if I remember my stat class correctly).
It is customary in science to reject the "null hypothesis" if the results of the measurements are less than 5% likely if the null hypothesis is true.
If a data distribution is approximately normal then about 68 percent of the data values are within one standard deviation of the mean (mathematically, μ ± σ, where μ is the arithmetic mean), about 95 percent are within two standard deviations (μ ± 2σ), and about 99.7 percent lie within three standard deviations
nuther fun fact
An intelligence quotient (IQ) is a total score derived from several standardized tests designed to assess human intelligence. ... By this definition, approximately two-thirds of the population scores are between IQ 85 and IQ 115
100 IQ IS the mean.
fifty percent of the population has an IQ lover than 100...fifty percent higher.
Apparently the "2/3 of the population" range depends upon both race and gender.
"An IQ gap of at least 1.1 SDs for American Blacks (average 24% White admixture)
and Whites has been present for the entire 100+ year history of IQ tests. The gap
between African Blacks and Whites is 2.0 SDs." (Radical Capitalism article)
That means that the mean IQ for African Blacks is within the lower 2.5% tail of the distribution for Whites.
I do intend to study the refereed article carefully. But if the Radical Capitalist article is summarizing it honestly, it means that the White Supremacists are actually scientifically correct. The possibility, and its implications, stun me.
I just noticed a second suspicious fact in your graph. According to the nonrefereed article, the IQ standard deviation for Asians is much lower than it is for whites. In your graph, the Asian standard deviation appears to be noticeably higher. (In your graph, the Asian curve is lower and broader than all of the other curves.)
I suspect that your graph comes from one particular study, while the refereed source that I cite is a survey article that reviews thirty years of research.
the IQ standard deviation for Asians is much lower than it is for whites
really?
In statistics, the standard deviation (SD, also represented by the Greek letter sigma σ or the Latin letter s) is a measure that is used to quantify the amount of variation or dispersion of a set of data values.[1] A low standard deviation indicates that the data points tend to be close to the mean (also called the expected value) of the set, while a high standard deviation indicates that the data points are spread out over a wider range of values.
Exactly. Here's another quote from the unrefereed Radical Capitalist article: "Asian IQ scores cluster around the mean; thus, the cognitive variation among Whites produces more geniuses, but also more morons."
in my not so humble and somewhat uneducated opinion (math is hard and I only took one stat class)
that is a non sensical statement.
perhaps there is good reason that the article was not refereed...they found math to be hard also...
I agree that they express the idea imprecisely, but if you read the quote in its context, the meaning is clear. Increase the standard deviation for a given mean and you would get both more geniuses and more morons. They are clearly claiming that the standard deviation for Asians is lower.
Such a claim is plausible on genetic grounds. I read that one out of five Asians have a common ancestor, according to their DNA, and that common ancestor is hypothesized to be Gengis Khan, who reportedly copulated with a different woman each day for decades of his reign.
Sampling his conquered population must certainly have been fun for Gengis Khan, and, as they say, "size does matter".
Slightly more seriously, any actual living population, say of Caucasians, is thought of as having been drawn independently, one at a time, from some imagined process such that the drawings are characterized by that formula for a normal probability density function.
If that is true (that the process really produces normally distributed drawings), then mathematics can be used to prove that the sample mean is an unbiased estimator of the population mean. It turns out that the "adjusted sample standard deviation", which is calculated using N-1 rather than N (where N is the sample size) is an unbiased estimator of the population standard deviation.
So you can get an unbiased estimate of the population standard deviation from a sample of any size with at least two observations, but of course the estimate will be more reliable for greater sample sizes.
Cite your source, please. That graph conflicts with the following, which claims that the overlap between the two distributions is small:
"The least intelligent 10% of Whites have IQs below 80 (low functioning) while 40% of Blacks fall below this level.
"Only one Black in six is more intelligent than the average White; five Whites out of six are more intelligent than the average Black.
"About 2.3% of Whites have an IQ of at least 130 (gifted), 20 times greater than the percentage of Blacks who do; only 0.00044% of African Blacks have an IQ over 130. 80% of gifted American Blacks have White admixture.
"Geniuses by Race (IQ 140 or higher):
• African Blacks 1:3,500,000 (0.000003%)
• American Blacks 1:218,000 (0.0004%)
• Whites 1:83 (1.2%)
"The per capita genius rate for Whites is 41,000 times higher than it is for African Blacks. If all Whites in America were replaced by Blacks, the number of geniuses in the country would fall from about 2.4 million to only about 1,000."
The above is clipped from the Radical Capitalist article, which cites the refereed article. I have not yet read the refereed article, which is 60 pages long, but plan to do so to verify that the other article is citing honestly.
But my question remains, WHAT IF? Rather than argue whether the difference exists, we should first confront what the implications would be if it is true and whether we are capable of admitting that it might be true. If we cannot admit that it might be true, then we cannot hope to be able to reason impartially about whether it is in fact true.
Rather than argue whether the difference exists, we should first confront what the implications would be if it is true and whether we are capable of admitting that it might be true
ya think? When has the truth ever mattered?
I was hoping for a serious discussion. I guess no one here has the guts to tackle this forbidden topic. :(
if the discussion doesn't go in the direction that you want it to then it's not serious?
ok..
gotcha.
Hey, give me the benefit of doubt! Remember, the standard deviation for whites is higher, which is why there are so many morons like me who can't understand your cryptic posts. :P
words mean things.
what does standard deviation mean?
is the bell curve taller or flatter?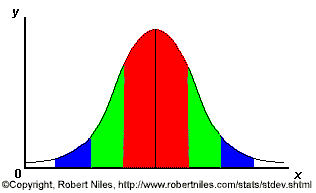
f
Ok. For the normal probability density function, it is the parameter that "means" the amount of variation around the mean. (See the sigma in the formula for a normal PDF here: https://en.wikipedia.org/wiki/Normal_distribution)
But I don't know what you are getting at? And it's probably going to take me a few days to find time to read the refereed article.
You edited your post to add the diagram and the second question after I responded to it. The most useful answer to your first question is that any normally distributed random variable has a probability of approximately 95% of being within two standard deviations of the mean (if I remember my stat class correctly).
It is customary in science to reject the "null hypothesis" if the results of the measurements are less than 5% likely if the null hypothesis is true.
Fun Fact:
nuther fun fact
100 IQ IS the mean.
fifty percent of the population has an IQ lover than 100...fifty percent higher.
Apparently the "2/3 of the population" range depends upon both race and gender.
"An IQ gap of at least 1.1 SDs for American Blacks (average 24% White admixture)
and Whites has been present for the entire 100+ year history of IQ tests. The gap
between African Blacks and Whites is 2.0 SDs." (Radical Capitalism article)
That means that the mean IQ for African Blacks is within the lower 2.5% tail of the distribution for Whites.
I do intend to study the refereed article carefully. But if the Radical Capitalist article is summarizing it honestly, it means that the White Supremacists are actually scientifically correct. The possibility, and its implications, stun me.
source
I just noticed a second suspicious fact in your graph. According to the nonrefereed article, the IQ standard deviation for Asians is much lower than it is for whites. In your graph, the Asian standard deviation appears to be noticeably higher. (In your graph, the Asian curve is lower and broader than all of the other curves.)
I suspect that your graph comes from one particular study, while the refereed source that I cite is a survey article that reviews thirty years of research.
the IQ standard deviation for Asians is much lower than it is for whites
really?
In statistics, the standard deviation (SD, also represented by the Greek letter sigma σ or the Latin letter s) is a measure that is used to quantify the amount of variation or dispersion of a set of data values.[1] A low standard deviation indicates that the data points tend to be close to the mean (also called the expected value) of the set, while a high standard deviation indicates that the data points are spread out over a wider range of values.
kinda like this?
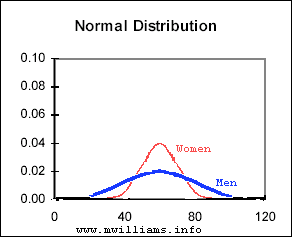
is it right to say that males are more likely to score better than females in math, when it's also true that they're more likely to score worse?
Exactly. Here's another quote from the unrefereed Radical Capitalist article: "Asian IQ scores cluster around the mean; thus, the cognitive variation among Whites produces more geniuses, but also more morons."
"Asian IQ scores cluster around the mean;
in my not so humble and somewhat uneducated opinion (math is hard and I only took one stat class)
that is a non sensical statement.
perhaps there is good reason that the article was not refereed...they found math to be hard also...
I agree that they express the idea imprecisely, but if you read the quote in its context, the meaning is clear. Increase the standard deviation for a given mean and you would get both more geniuses and more morons. They are clearly claiming that the standard deviation for Asians is lower.
Such a claim is plausible on genetic grounds. I read that one out of five Asians have a common ancestor, according to their DNA, and that common ancestor is hypothesized to be Gengis Khan, who reportedly copulated with a different woman each day for decades of his reign.
math is fun
just guessing..but it would seem that the size of the population matters.
Sampling his conquered population must certainly have been fun for Gengis Khan, and, as they say, "size does matter".
Slightly more seriously, any actual living population, say of Caucasians, is thought of as having been drawn independently, one at a time, from some imagined process such that the drawings are characterized by that formula for a normal probability density function.
If that is true (that the process really produces normally distributed drawings), then mathematics can be used to prove that the sample mean is an unbiased estimator of the population mean. It turns out that the "adjusted sample standard deviation", which is calculated using N-1 rather than N (where N is the sample size) is an unbiased estimator of the population standard deviation.
So you can get an unbiased estimate of the population standard deviation from a sample of any size with at least two observations, but of course the estimate will be more reliable for greater sample sizes.